This Thursday is Pi Day, the one day of the year devoted to a mathematical constant (it also happens to be Einstein’s birthday). We all learned as kids about how special pi is and have since accepted its cultural status as a geeky totem and a number worth celebrating. But perhaps we should revisit pi through the jaded lens of adulthood. The circumference of a circle is always 3.14… times its diameter. Big whoop! The perimeter of a square is four times its side length, but we don’t assign special significance to the number four or bake square desserts on April 4 or hold contests for memorizing the digits of four. So why all the fuss about pi?
I’ll attempt to show rather than tell an answer to that question, using two beautiful pi-centric puzzles. Even if you find them easy as pie, I hope they renew your childlike enthusiasm for the little constant.
Did you miss last week’s puzzle? Check it out here, and find its solution at the bottom of today’s article. Be careful not to read too far ahead if you haven’t solved last week’s yet!
Puzzle #33: Pi Day
- A string is tightly wrapped around Earth’s equator. You splice additional string in to add just enough slack so that you could (in principle) raise the new longer string exactly 1 foot off the ground all around the world. How much string did you add? How much would you need to add to a string wrapped around a basketball to raise it by 1 foot?
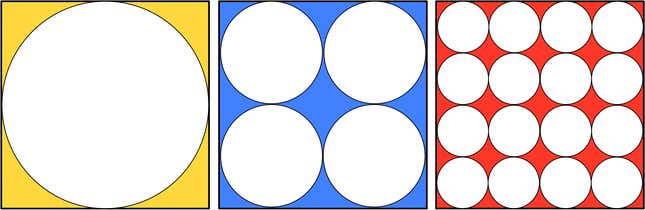
- In the image below, which area is the largest, yellow, blue, or red? The squares are all the same size and all of the circles within the same square have equal size. The circles just barely kiss each other and the edges of the squares at single points.
I’ll be back next Monday with the answers and a new puzzle. Do you know a cool puzzle that you think should be featured here? Message me on X @JackPMurtagh or email me at gizmodopuzzle@gmail.com
Solution to Puzzle #32: Forehead or Dead
Did you escape your captor’s clutches in last week’s puzzle? I rank this among the hardest Gizmodo Monday Puzzles yet. I was heartened to see some teamwork in the comments section. Eugenius described the correct scheme and Alfred-WhyDidYouSayThatName tidied up the idea with the right mathematical notation. Well done!
The solution involves remainders when dividing. For a little remainder reminder, remember that 20 divided by 10 has remainder 0, because 10 goes into 20 evenly, whereas 23 divided by 10 has remainder 3, because there are 3 left over after the division.
You only know nine of the 10 cards (all but your own). The key insight is that if you also knew the remainder when you sum all 10 cards and divide that sum by 10, then you could deduce your own card. For example, imagine you knew that summing all 10 cards and dividing the result by 10 would yield a remainder of 0 (i.e., the sum of all 10 cards is divisible by 10). You can see nine of the cards, so you add them up and get 63. Now you know your card must be a 7, because that’s the only available number that, when added to 63, results in a number divisible by 10. This type of reasoning works no matter what the remainder is. If you had been told that the remainder is 1 and the cards you saw added to 63, then you would know that yours was an 8.
In practice, you don’t have this extra remainder information, but the above example shows that if you did, then you could deduce your own card. To solve the puzzle, the group assigns each prisoner a different number from 0 to 9 to represent all possible remainders. Each person acts as though their assigned number is the correct remainder when all 10 cards are summed and divided by 10. One of these people must be correct because all possible remainders are accounted for.
You might wonder how on Earth you were supposed to realize that remainders were the key concept. Given the many possible distributions of cards, the group needs to somehow sort all of the cases into only 10 categories so that each prisoner can handle one of the categories. I’m not saying it’s easy, but after some experimentation with smaller groups of people, remainders eventually emerge as a natural way to sort the possibilities.






